I’m currently in the middle of reading Sam Harris’ new book, The Moral Landscape: How Science Can Determine Human Values. I had to take a few minutes to pause and look up something he mentioned, called The Monty Hall problem. Here is part of the Wikipedia article on this:
The Monty Hall problem is a probability puzzle loosely based on the American television game show Let’s Make a Deal. The name comes from the show’s original host, Monty Hall. The problem is also called the Monty Hall paradox, as it is a veridical paradox in that the result appears absurd but is demonstrably true.
The problem was originally posed in a letter by Steve Selvin to the American Statistician in 1975. A well-known statement of the problem was published in Marilyn vos Savant‘s “Ask Marilyn” column in Parade magazine in 1990:
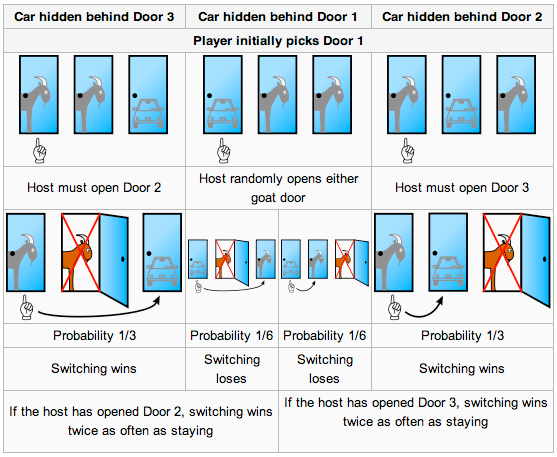
Suppose you’re on a game show, and you’re given the choice of three doors: Behind one door is a car; behind the others, goats. You pick a door, say No. 1, and the host, who knows what’s behind the doors, opens another door, say No. 3, which has a goat. He then says to you, “Do you want to pick door No. 2?” Is it to your advantage to switch your choice?Although not explicitly stated in this version, solutions are almost always based on the additional assumptions that the car is initially equally likely to be behind each door and that the host must open a door showing a goat, must randomly choose which door to open if both hide goats, and must make the offer to switch.
As the player cannot be certain which of the two remaining unopened doors is the winning door, and initially all doors were equally likely, most people assume that each of two remaining closed doors has an equal probability and conclude that switching does not matter; hence the usual answer is “stay with your original door”. However, under standard assumptions, the player should switch—doing so doubles the overall probability of winning the car from 1/3 to 2/3.
The Monty Hall problem, in its usual interpretation, is mathematically equivalent to the earlier Three Prisoners problem, and both bear some similarity to the much older Bertrand’s box paradox. These and other problems involving unequal distributions of probability are notoriously difficult for people to solve correctly; when the Monty Hall problem appeared in Parade, approximately 10,000 readers, including nearly 1,000 with PhDs, wrote to the magazine claiming the published solution (“switch!”) was wrong. Numerous psychological studies examine how these kinds of problems are perceived. Even when given a completely unambiguous statement of the Monty Hall problem, explanations, simulations, and formal mathematical proofs, many people still meet the correct answer with disbelief.
Harris explains how, even when presented with the facts and eventually understanding why this is logically true, we still have a hard time shaking our initial reaction that falsely thought staying at the original door would have the same odds as switching. He uses this to illustrate that it is a fault in human reasoning, not a fault in the actual answer. And while it may be tricky for us to understand at first what is the correct answer, it doesn’t mean that there is not a true answer. And this often happens with certain questions on what is moral and what is not. He explains that just because a certain issue has opposing opinions or is one of which most people agree is hard to figure out the answer, it does not mean that an answer does not exist when it comes to certain questions of ethics. (He goes a lot deeper into this in the book).
Part of me feels foolish for not originally knowing the correct answer. It seems pretty obvious after it’s been explained. But then I go back and then think, “Wait. No, my original answer was right. I don’t get it”. Seeing this visual explanation helped it sink in my head more.
I’m almost 2/3 finished with this book. It’s been hard to put down. It’s really fascinating to hear morality explained from a scientific, neurological point of view. I won’t be able to fully decide my opinion on this book overall until I have completely read it, but I know so far that I’ve already learned a lot of things and am looking forward to his speaking engagement in DC next week.
